From the LISA proposal:

First order thermal phase transition:

1. Bubbles nucleate and grow
2. Expand in a plasma - create shock waves
3. Bubbles + shocks collide - violent process
4. Sound waves left behind in plasma
5. Turbulence; expansion

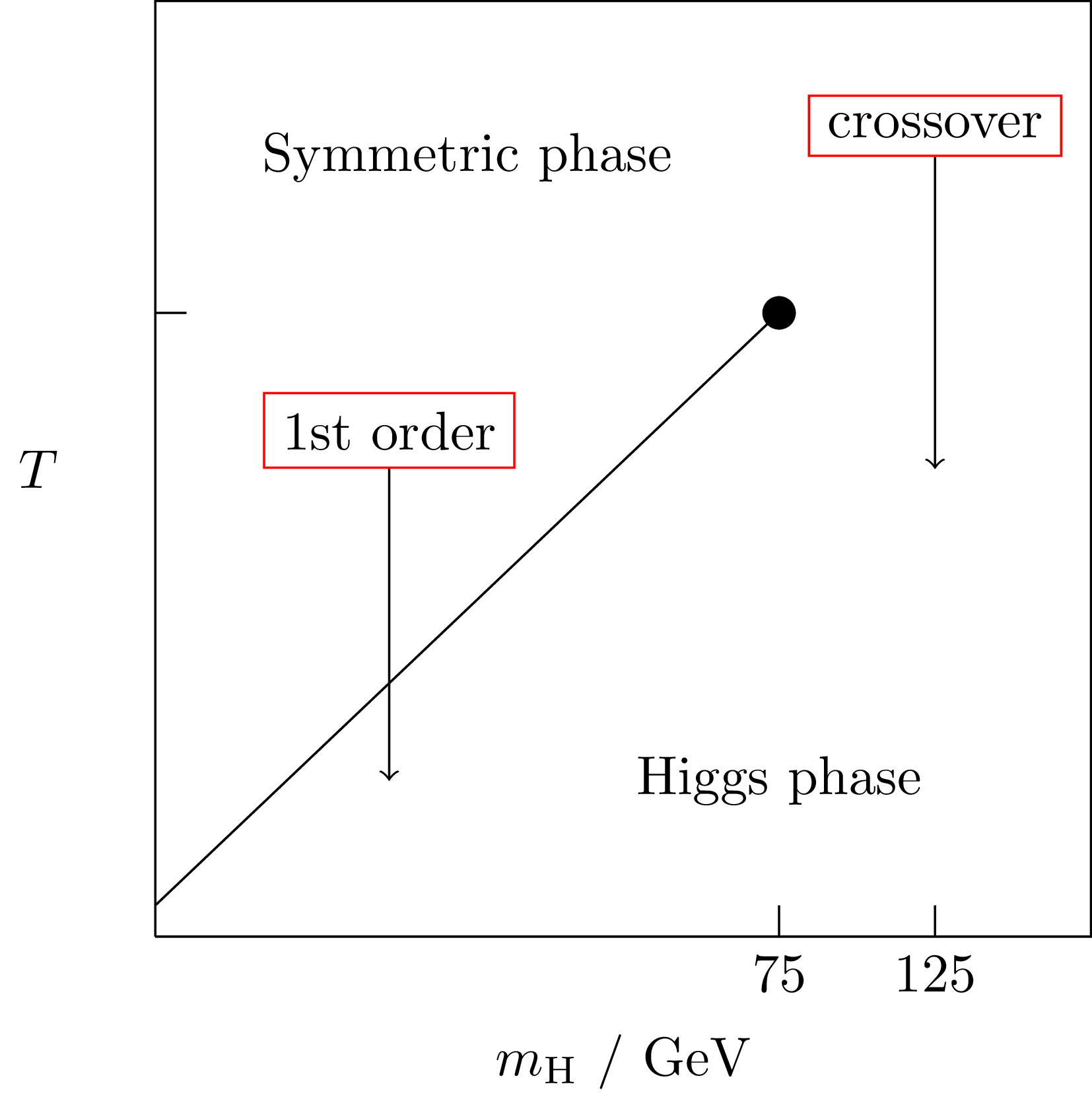
Thermal phase transitions

• Standard Model is a crossover
  Kajantie et al.; Karsch et al.; ...
• First order possible in extensions (xSM, 2HDM, ...)
  Andersen et al., Kozaczuk et al., Carena et al.,
  Bödeker et al., Damgaard et al., Ramsey-Musolf et al.,
  Cline and Kainulainen, ...
• Baryogenesis?
• GW PS ⇔ model information?

What the metric sees at a thermal phase transition

• Bubbles nucleate and expand, shocks form, then:
  1. $h^2 \Omega_\phi$: Bubbles + shocks collide - 'envelope phase'
  2. $h^2 \Omega_\text{sw}$: Sound waves set up - 'acoustic phase'
  3. $h^2 \Omega_\text{turb}$: [MHD] turbulence - 'turbulent phase'


• Sources add together to give observed GW power: $$ h^2 \Omega_\text{GW} \approx h^2 \Omega_\phi + h^2 \Omega_\text{sw} + h^2 \Omega_\text{turb}$$

Coupled field and fluid system

• Scalar $\phi$ and ideal fluid $u^\mu$:
  • Split stress-energy tensor $T^{\mu\nu}$ into field and fluid bits $$\partial_\mu T^{\mu\nu} = \partial_\mu (T^{\mu\nu}_\phi + T^{\mu\nu}_\text{fluid}) = 0$$
  • Parameter $\eta$ sets the scale of friction due to plasma $$\partial_\mu T^{\mu\nu}_\phi = \tilde \eta \frac{\phi^2}{T} u^\mu \partial_\mu \phi \partial^\nu \phi $$
  • $V(\phi,T)$ is a 'toy' potential tuned to give latent heat $\mathcal{L}$
  • $\beta$ ↔ number of bubbles; $\alpha_{T_*}$ ↔ $\mathcal{L}$, $v_\text{wall}$ ↔ $\tilde \eta$

Begin in spherical coordinates:
what sort of solutions does this system have?

Velocity profile development: small $\tilde \eta$ ⇒ detonation (supersonic wall)

Velocity profile development: large $\tilde \eta$ ⇒ deflagration (subsonic wall)

Velocity power spectra and power laws

Fast deflagration

Detonation

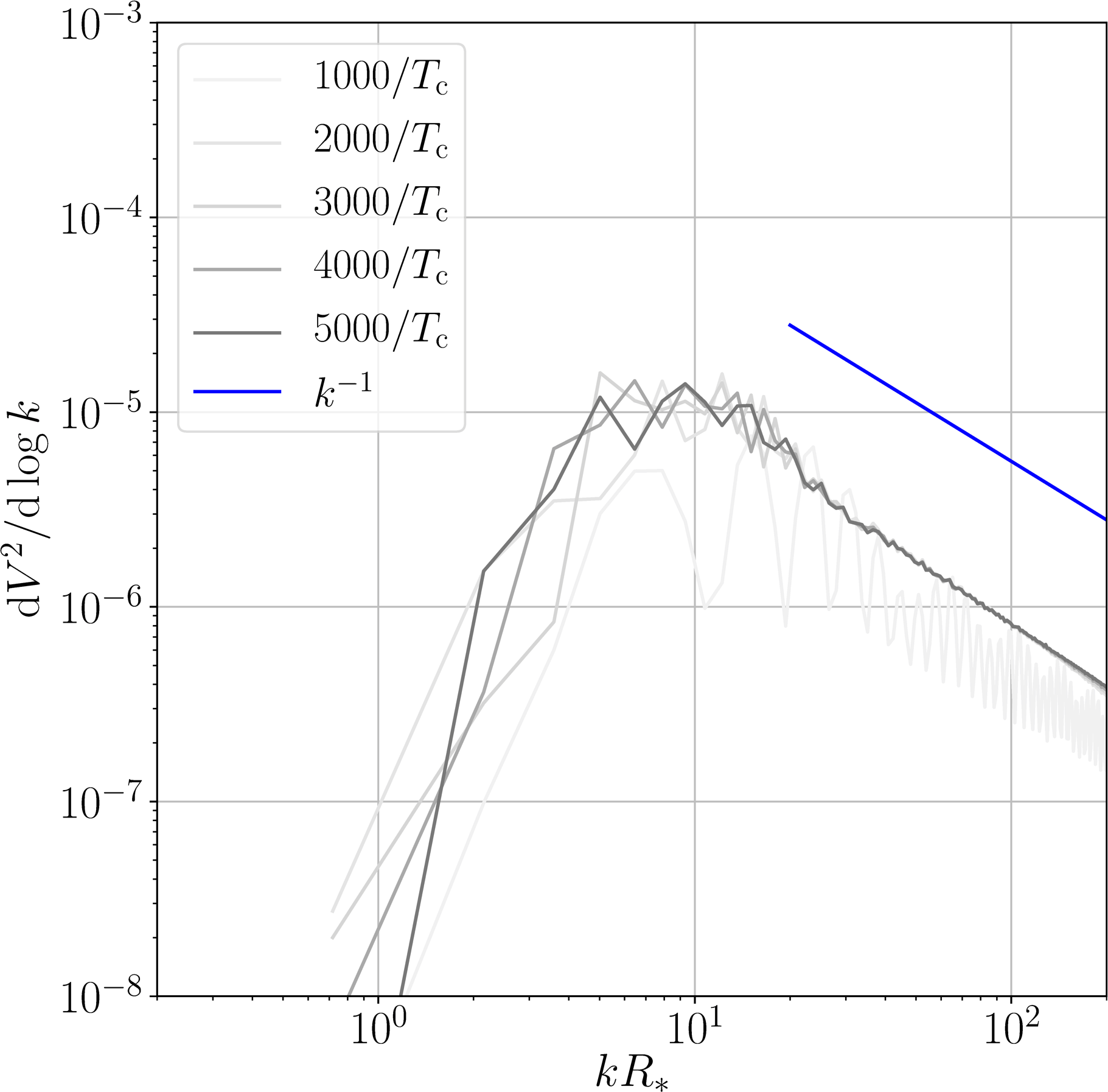
• Weak transition: $\alpha_{T_*} =0.01$
• Power law behaviour above peak is between $k^{-2}$ and $k^{-1}$
• “Ringing” due to simultaneous nucleation, unimportant

GW power spectra and power laws

Fast deflagration

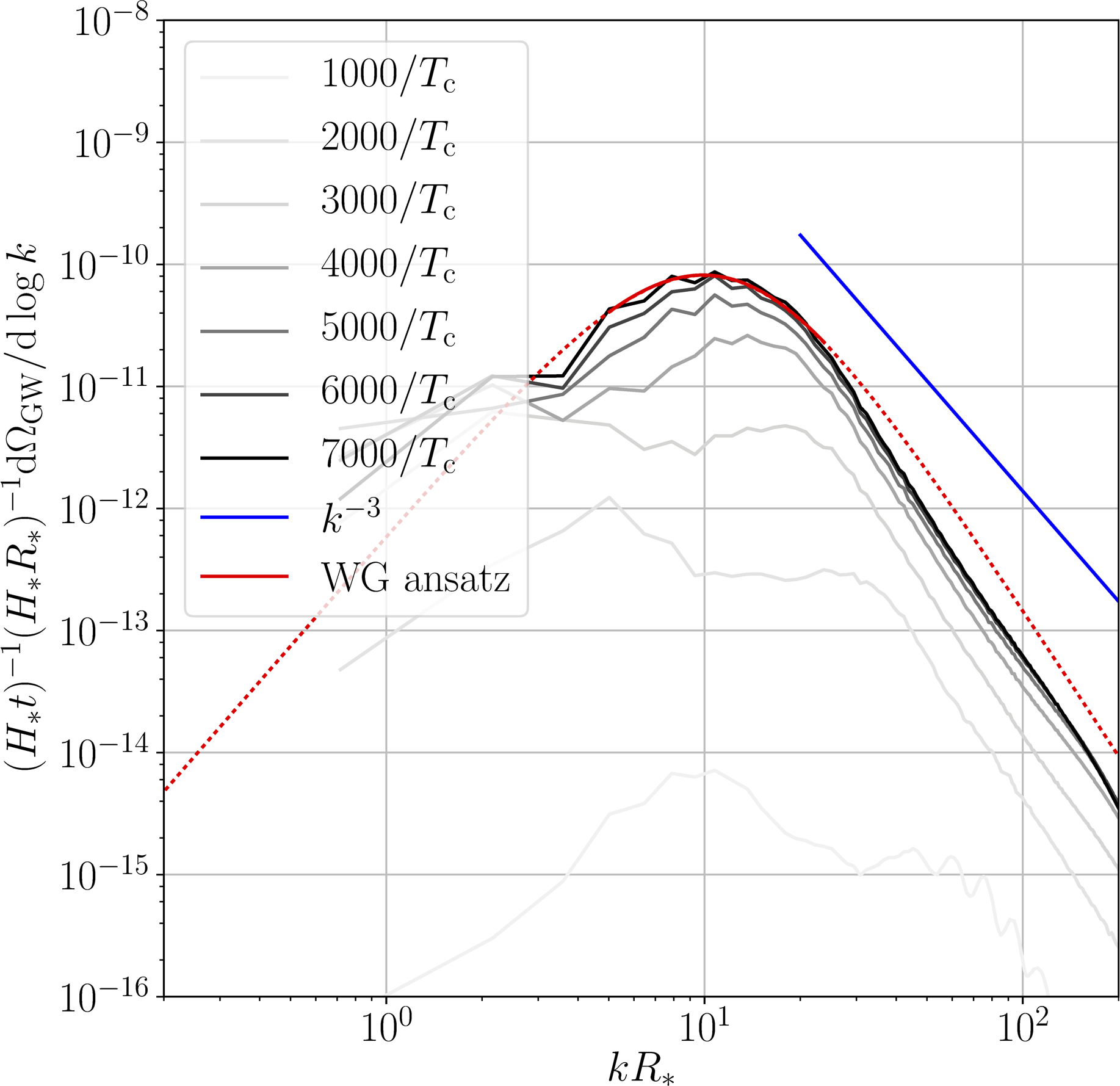
Detonation

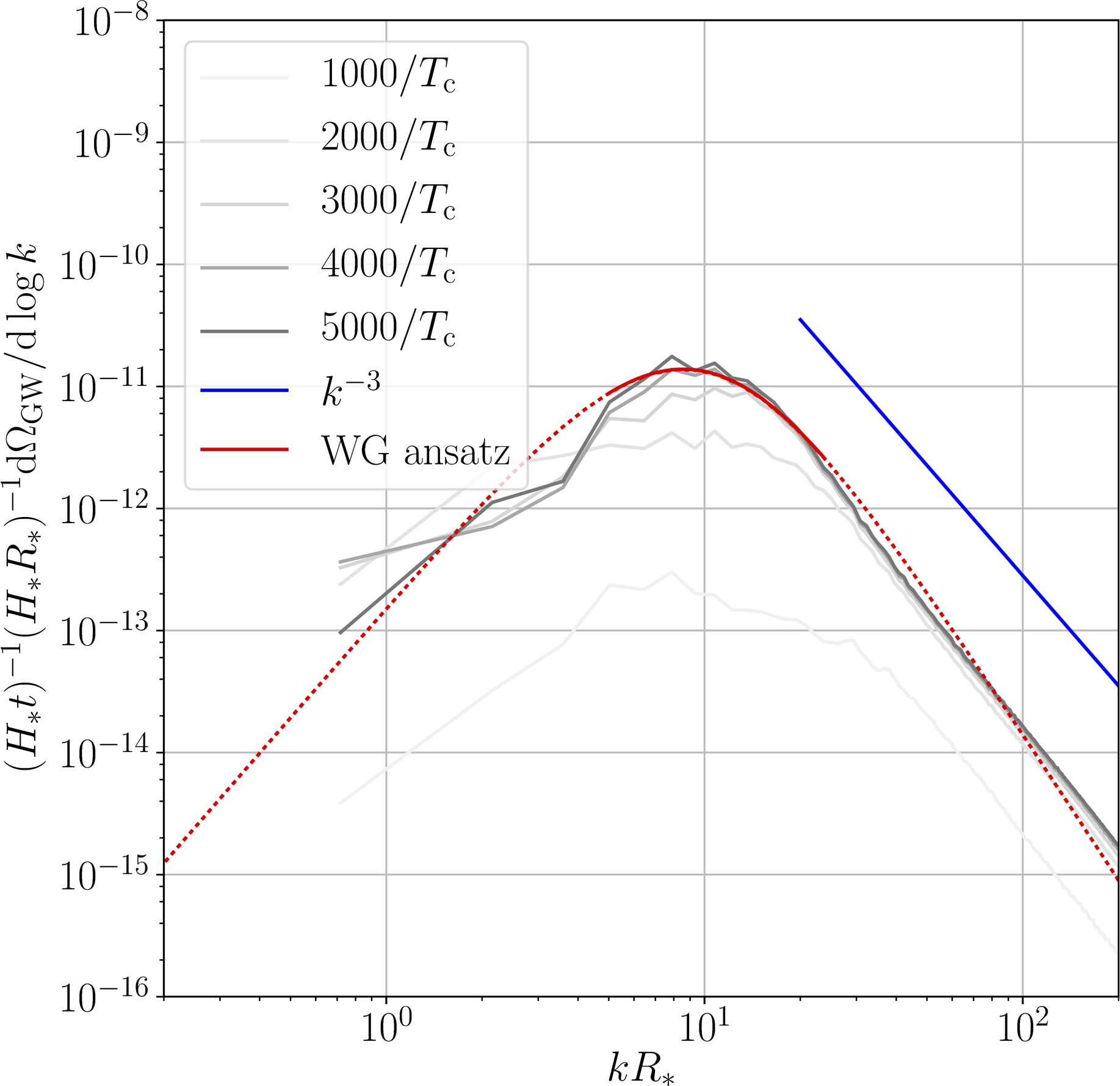
• Causal $k^3$ at low $k$, approximate $k^{-3}$ or $k^{-4}$ at high $k$
• Curves scaled by $t$: source until turbulence/expansion

→ power law ansatz for $h^2 \Omega_\text{sw}$

Transverse versus longitudinal modes – turbulence?

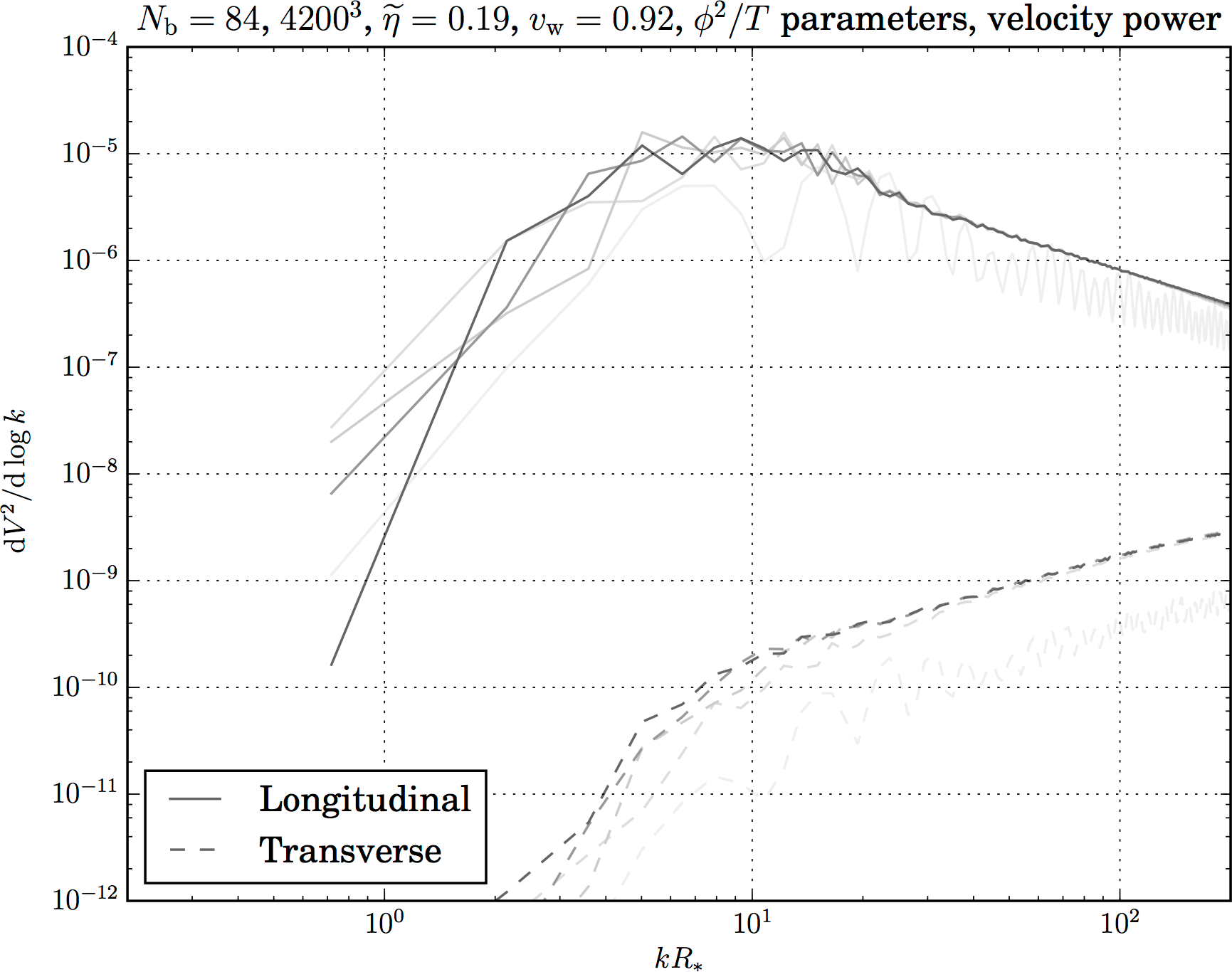
• Short simulation; weak transition (small $\alpha$): linear; most power in longitudinal modes ⇒ acoustic waves, turbulent
• Turbulence requires longer timescales $R_*/\overline{U}_\mathrm{f}$
• Plenty of theoretical results, use those instead
  Kahniashvili et al.; Caprini, Durrer and Servant; Pen and Turok; ...

→ power law ansatz for $h^2 \Omega_\text{turb}$

Putting it all together - $h^2 \Omega_\text{gw}$ arXiv:1512.06239

• Three sources, $\approx$ $h^2\Omega_\phi$, $h^2\Omega_\text{sw}$, $h^2 \Omega_\text{turb}$
• Know their dependence on $T_*$, $\alpha_T$, $v_\mathrm{w}$, $\beta$
  Espinosa, Konstandin, No, Servant
• Know these for any given model, predict the signal...

(example, $T_* = 100 \mathrm{GeV}$, $\alpha_{T_*} = 0.5$, $v_\mathrm{w} =0.95$, $\beta/H_* = 10$)

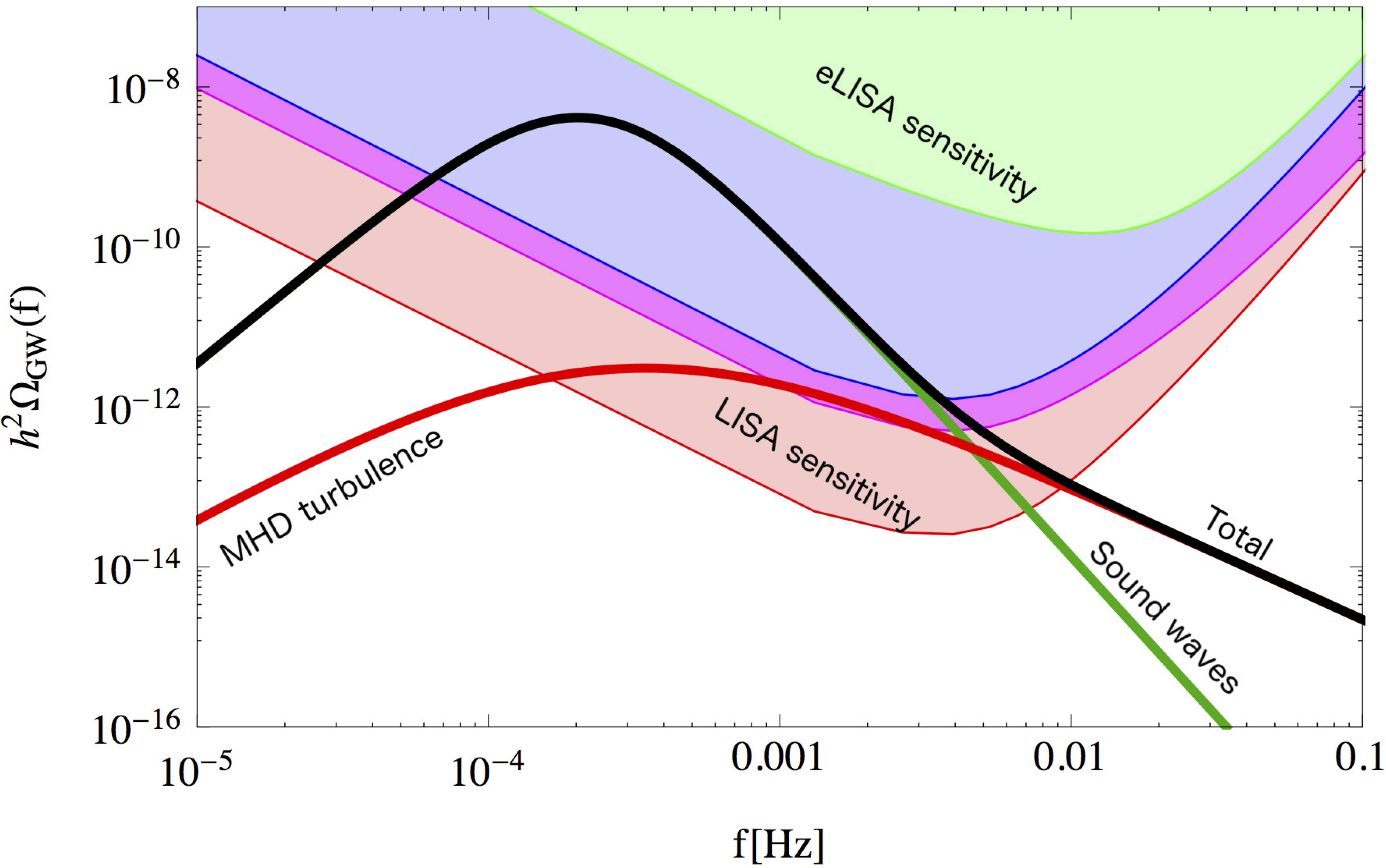
Putting it all together - physical models to GW power spectra

Model ⟶ ($T_*$, $\alpha_{T_*}$, $v_\mathrm{w}$, $\beta$) ⟶ this plot

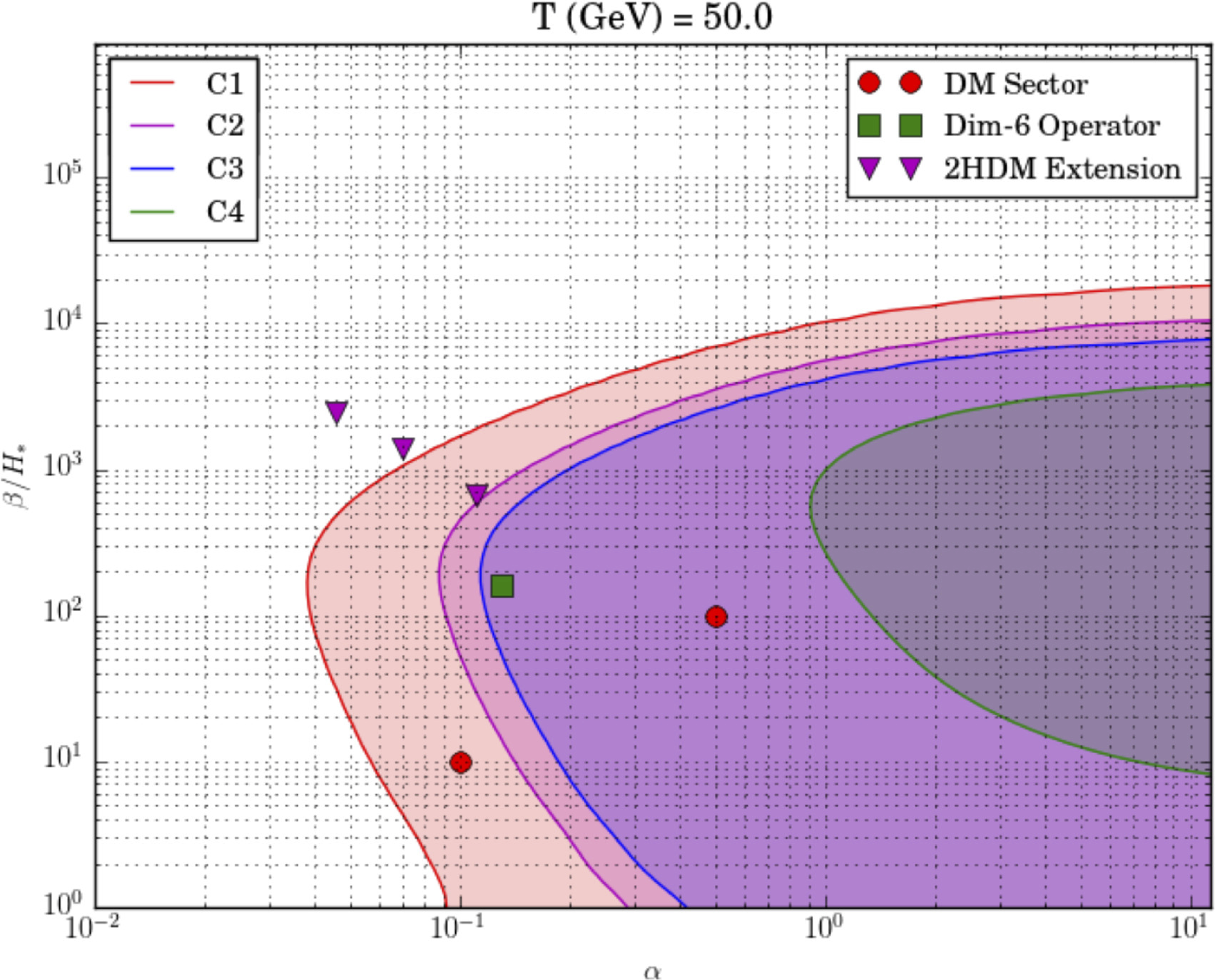
... which tells you if it is detectable by LISA (see arXiv:1512.06239)

Sensitivity of LISA to acoustic waves alone

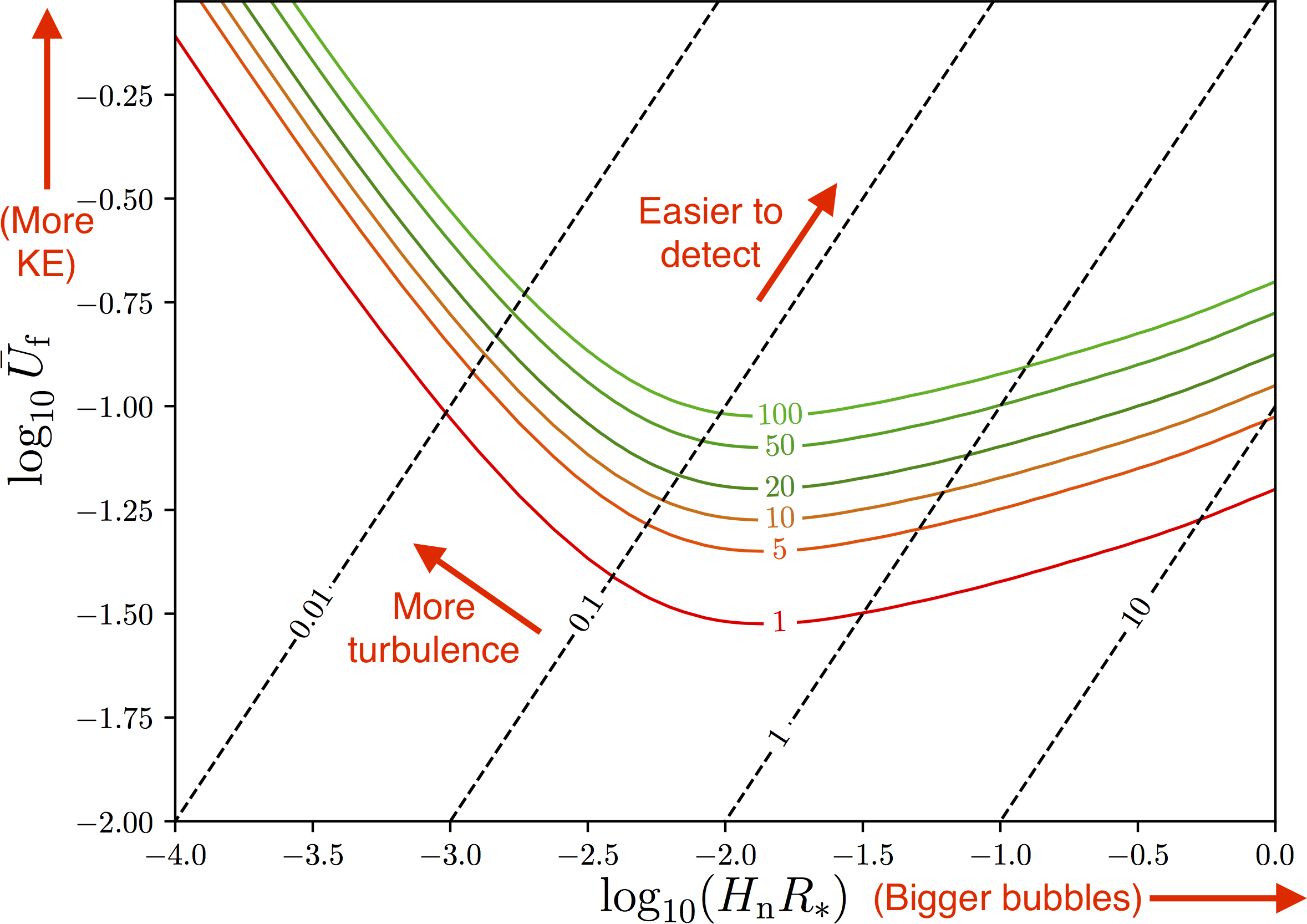
The pipeline